Freefall From Specified Height
For an object falling from rest through air with quadratic drag, the motion parameters are usually expressed in terms of the terminal velocity and the characteristic time: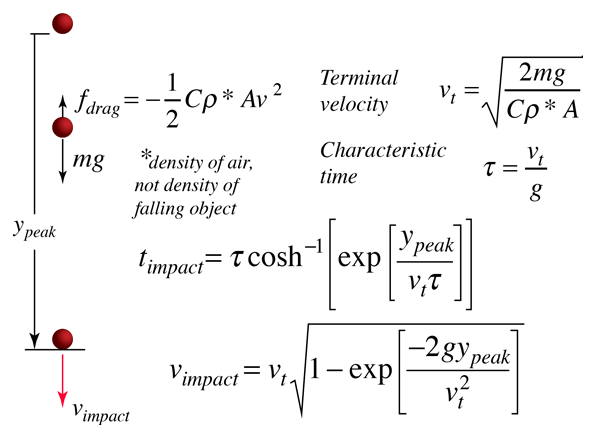
Note: A value of g = 9.8 m/s2 is presumed in the above calculation.
| Hyperbolic functions |
Fluid friction
| HyperPhysics***** Mechanics ***** Fluids | R Nave |