Conduction Electron Population for Semiconductor
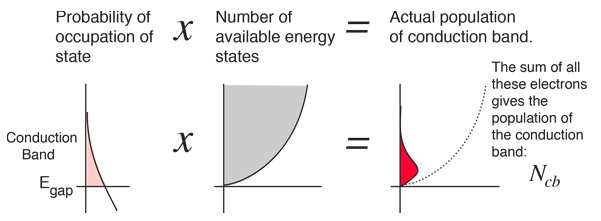
The conduction electron population for a semiconductor is calculated by multiplying the density of conduction electron states r(E) times the Fermi function f(E). The number of conduction electrons as a function of energy is then given by
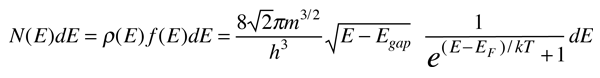
This can be simplified by noting that for the energies of the conduction band, E-EF>>1, so the 1 in the denominator of the Fermi function becomes insignificant. I.e., the tail of the function which extends into the conduction band is so far out that it can be approximated by the Boltzmann function. Using the fact that
The population density can then be written
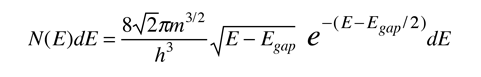
The total number of electrons in the conduction band, Ncb, can then be obtained by integrating the above function from the bottom of the conduction band upward. For all practical purposes, the upper limit of the integral can be taken to be infinity since by the time we reach the top of the conduction band, the integrand will be essentially zero.
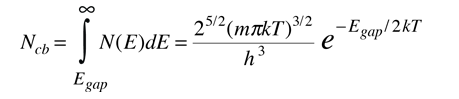 |
|
Semiconductor concepts
Semiconductors for electronics
Reference
Simpson
Sec 4.7
| HyperPhysics***** Condensed Matter ***** Electricity and Magnetism | R Nave |