Energy and Momentum Spectra for Beta Decay

Beta emission has a characteristic energy spectrum. It is accompanied by the emission of an electron antineutrino which shares the momentum and energy of the decay. This experimental energy spectrum is from G. J. Neary, Roy. Phys. Soc. (London), A175, 71 (1940).

From the Fermi theory of beta decay, the shape of the energy distribution for this "allowed" transition is given approximately by the expression

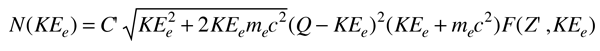
where F(Z',KE_e) is called the Fermi function. It accounts for the nuclear coulomb interaction which shifts this distribution toward lower energies because of the coulomb attraction between the daughter nucleus and the emitted electron. (It shifts the distribution upward for positrons.) Q represents the energy yield of the transition and as such is the upper bound on the kinetic energy of the electron, KE_e. The apparent complexity of the expression is partly because it is necessary to use relativistic momentum for the electron.

The emission of the electron's antiparticle, the positron, is also called beta decay.

The nucleus ⁶⁴Cu is a good example for momentum spectra since it decays by both electron (to ⁶⁴Zn) and positron emission (to ⁶⁴Ni) with comparable transition energies. The experimental spectra below are from Cook and Langer, Phys. Rev. 73, 601 (1948) with theoretical refinement by J. R. Reitz, Phys. Rev. 77, 10 (1950). This allows you to see clearly that the momentum spectrum of the positron is shifted toward higher momentum by the coulomb repulsion of the nucleus.

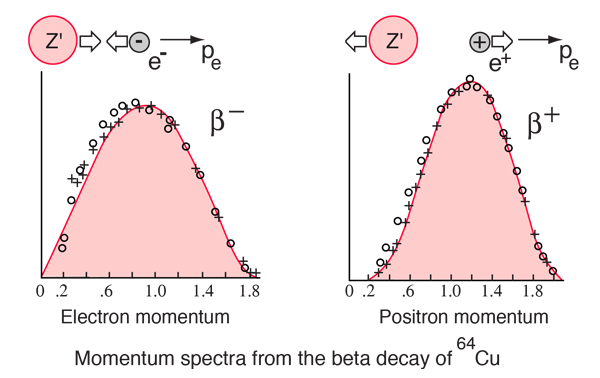
From the Fermi theory of beta decay, the shape of the distributions for these "allowed" transitions is given approximately by the expression

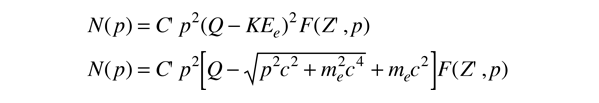
where F(Z',p) is called the Fermi function. It accounts for the nuclear coulomb interaction and is the main source of the difference between the two curves above. Again, the expression looks a bit complex because the relativistic relationship between momentum and enegy must be used for beta decay.