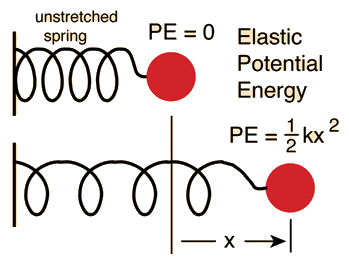
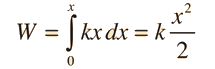Elastic Potential Energy
Elastic potential energy is Potential energy stored as a result of deformation of an elastic object, such as the stretching of a spring. It is equal to the work done to stretch the spring, which depends upon the spring constant k as well as the distance stretched. According to Hooke's law, the force required to stretch the spring will be directly proportional to the amount of stretch.|
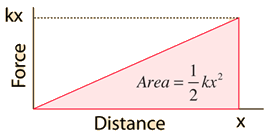
Since the force has the form F = -kxthen the work done to stretch the spring a distance x is 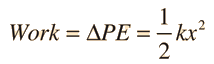 Show details |
|
Energy concepts
| HyperPhysics***** Mechanics | R Nave |