Complex Numbers
A complex number is a number with a real and an imaginary part, usually expressed in cartesian form
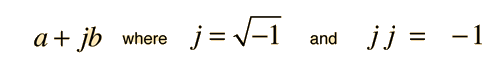
The polar form can also be expressed in terms of trigonometric functions using the Euler relationship
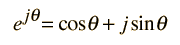
Complex numbers
| HyperPhysics****HyperMath*****Algebra | R Nave |



