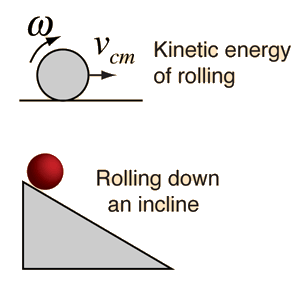
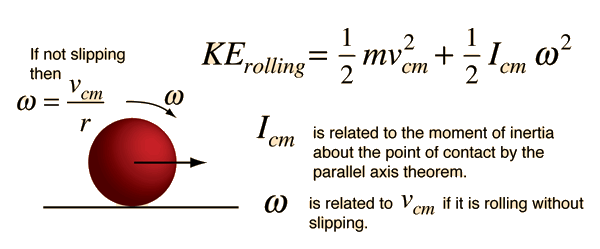Work-Energy Principle
The work-energy principle is a general principle which can be applied specifically to rotating objects. For pure rotation, the net work is equal to the change in rotational kinetic energy:

For a constant torque, the work can be expressed as
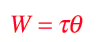
and for a net torque, Newton's 2nd law for rotation gives

Combining this last expression with the work-energy principle gives a useful relationship for describing rotational motion.
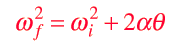
Rotation concepts
Rotational kinetic energy concepts
| HyperPhysics***** Mechanics ***** Rotation | R Nave |